Time Value and Intrinsic Value

The most important variable you need to understand is the fluctuating nature of the value of time and the continual erosion of its value each passing day. Time loses value faster as an option approaches its expiration. For an option to be profitable on expiration, any loss of time value must be recouped by an increase in the option’s intrinsic value.

The time value represents a mathematical calculation about how much the underlying could move given the amount of time left before expiration. This mathematical estimate includes everything that goes into pricing an option except its intrinsic value. Option market makers refer to this combination of factors as implied volatility. We’ve referred to it as time value. Whatever you choose to call it, remember that it can fluctuate and become more or less expensive due to market conditions, that it decays in value over time, and that it always works against an option buyer and for an option seller.

Conversely the intrinsic value of an option is easy to determine. Simply compare the option’s strike price to its underlying’s price. It will always be either positive or zero. Call options (right to buy) have intrinsic value when the strike price is lower than the current price because you have the right to buy the stock for less than its current price. Put options (right to sell) have intrinsic value when the strike price is higher than the current price because they give you the right to sell the equity for higher than its current price. Options are usually displayed in tables (known as chains) with different color schemes making it easy to see which options have intrinsic (in-the-money) value and extrinsic (out-of-the-money) value.

For example, if a Call option gave you the right to buy the stock at a strike price of $40, and the equity currently trades at $42.23, then your option would have an intrinsic value of $2.23 ($42.23 current price – $40 right to buy price = $2.23 of intrinsic value). If the option cost $5.43, then time value would be $3.20 ($5.43 price of the option – $2.23 intrinsic value = $3.20 time value). On the other hand, a put option in this example would have zero intrinsic value ($40 right to sell price – $42.23 current price = -$2.23…a negative intrinsic value is automatically set to zero). If the $40 put option cost $3.20, the entire price is time value since the put option is out-of-the-money.

If the equity increases in price to $45 at expiration, the intrinsic value increases exactly as you might expect, penny for penny; while the time portion of the option’s price would continue to fluctuate based on market conditions and also constantly erode until expiration when it reaches zero. In this example, the $45 equity price at expiration is $5 higher than the $40 strike price of the call option. This call option has an intrinsic value of $5 which means the option ended up losing $0.43 at expiration because $5.43 original cost – $5 current value = $0.43 loss.

The only way for this (or any) option to be profitable on expiration is for the in-the-money value to move more than the amount of time value lost to time decay (in this case $3.20).

Options can be bought and sold at any time, it is not necessary to wait until expiration before selling a profitable option. In fact, it is often better not to wait until expiration before liquidating your option positions. Using this losing trade as an example, it is very possible that the equity moved around enough before all of the time value decayed for this option to have been profitable at some point during its lifespan.

Changes in Time value vs Intrinsic Value

If the underlying moves above the strike price of a call option by $1.00 over the course of a few days, the intrinsic value increases by $1.00, but the time value decreases because of the passage of time and fluctuating needs of the market makers. Awareness of how much is paid for the time portion of an option gives you a sense of how far the underlying must move in the desired direction for the option to be profitable on expiration. The following figure demonstrates how the price of a call option both fluctuates and decays over time in comparison to its underlying’s price. The intrinsic value is depicted in green, while the time value is depicted in red.

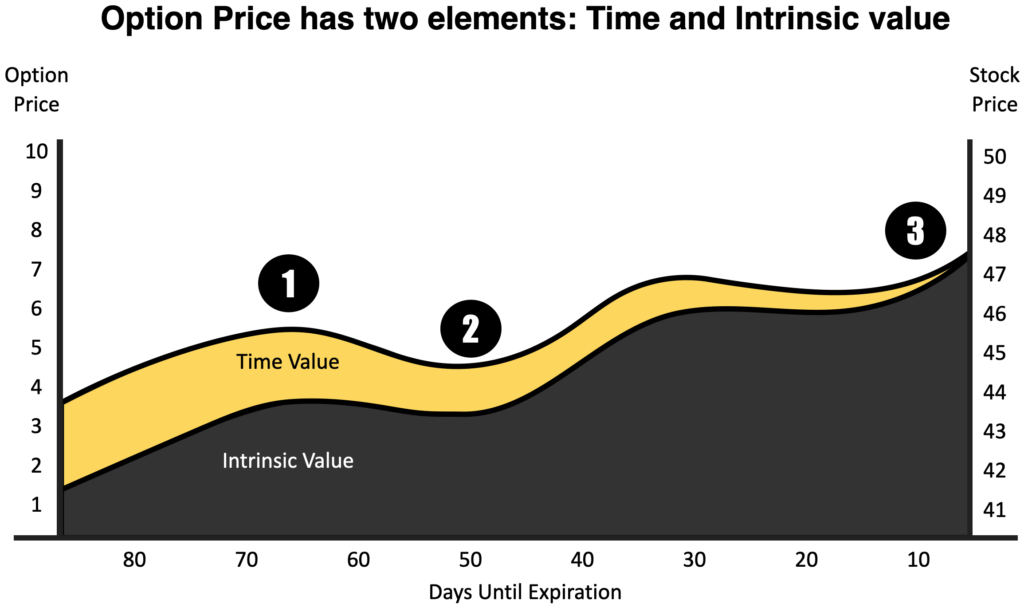
In this 90 day hypothetical example, the stock starts at $41.75 and fluctuates upward until it closes at $47.75 on the expiration date of the $40 call option also depicted. The initial price of the option is $3.75 of which $1.75 is intrinsic value (in dark gray) and $2.00 is time value (in yellow).

This chart demonstrates how the stock and option prices vary in a related but not exact fashion over time. Notice four important concepts shown in this figure:

1. Over the course of 90 days, the time value—depicted in yellow—diminishes from $2.00 until it reaches zero on expiration. (Note this decay is not linear.)
2. During those times the stock price trends flat, the option price will fall relative to the stock because of the affect of time decay as depicted between point 1 and 2
3. Towards the end of expiration, as depicted near point 3, the option price moves in close tandem to the stock price because the time value is closer to zero so a greater percentage of the option’s price is intrinsic value which moves penny for penny with the stock.
4. Unexpected volatility to the underlying can inflate the time portion of an option’s price though time decay always eats away at value as shown between points 2 and 3.

Understand that the time portion of an option’s price is influenced by both the passage of time and the rise and fall of implied volatility (or the effort by the market makers to insure a 70% winning percentage for themselves). Keeping this in mind helps you make better options trading decisions. As we’ll show in future instruction, there are a number of different strategies for trading options which take advantage of this central truism about the value of time.